Ferrotoroidic Order

Domains are of tremendous technological importance as they are used for information storage and for electronic, magnetic or optical switches. They are an essential property of any ferroic material. Three forms of ferroic order namely ferromagnetism (a spontaneous magnetisation), ferroelectricity (a spontaneous polarisation) and ferroelasticity (a spontaneous strain) are widely known.

When we sort them with respect to their symmetry properties we find that each of these belongs into its own class. However, as figure 1 shows, four of these classes exis. Extending the rationale, a novel type of ferroic order violating space- and time-inversion symmetry with a single order parameter should exist. This existence is fundamental and the inherent magnetoelectric coupling is technologically interesting.

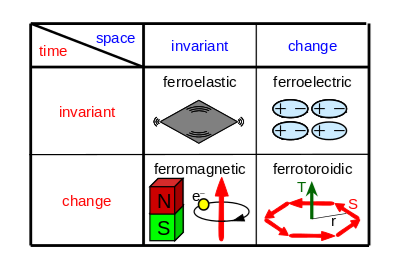
Fig 1: Forms of ferroic order with different behaviour under parity operations of space and time.

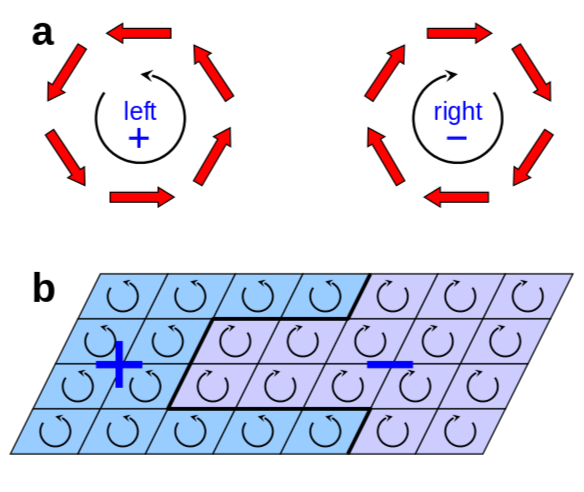
A uniform vortex-like alignment of magnetic moments, called ferrotoroidicity, was proposed to represent this state. Aside from the beauty of the symmetry achieved by introducing ferrotoroidicity as fourth form of ferroic order, the novel type of order has a link to device applications because the inherent magnetoelectricity, allows one to control magnetizations by electric fields and vice versa. In a variety of experiments we are further exploring this exciting new state of matter with a focus on the following issues:

Identification of new types of ferrotoroidic materials with a focus on highly engineerable toroidic ordered two-dimensional metamaterials.
Demonstration of ferrotoroidic poling in toroidal fields. Observing a poling hysteresis is a hallmark of any ferroic state and therefore an important step toward establishing this new type of ferroic order. Toroidal fields are conveniently generated by crossed magnetic and electric fields.
Quantification of the magnetoelectric coupling associated to the ferrotoroidic order.
Development of a comprehensive concept for the relation between the magnetoelectric effect, toroidal moments and magnetic monopoles.

Enlarged view: Fig 2: Ferrotoroidic order and domains. (a) Example for a right- and left-handed toroidal moment formed by the magnetic moments in a unit cell. (b) Schematic of ferrotoroidic domains (+,-) in a crystal. — Fig 2: Ferrotoroidic order and domains. (a) Example for a right- and left-handed toroidal moment formed by the magnetic moments in a unit cell. (b) Schematic of ferrotoroidic domains (+,-) in a crystal.

Reference

A. Zimmermann, D. Meier & M. Fiebig: Ferroic nature of magnetic toroidal order, Nature Communications 5, 4796 (2014)
N. A. Spaldin, M. Fiebig & M. Mostovoy: The toroidal moment in condensed-matter physics and its relation to the magnetoelectric effect, J. Phys.: Condens. Matter 20, 434203 (2008)